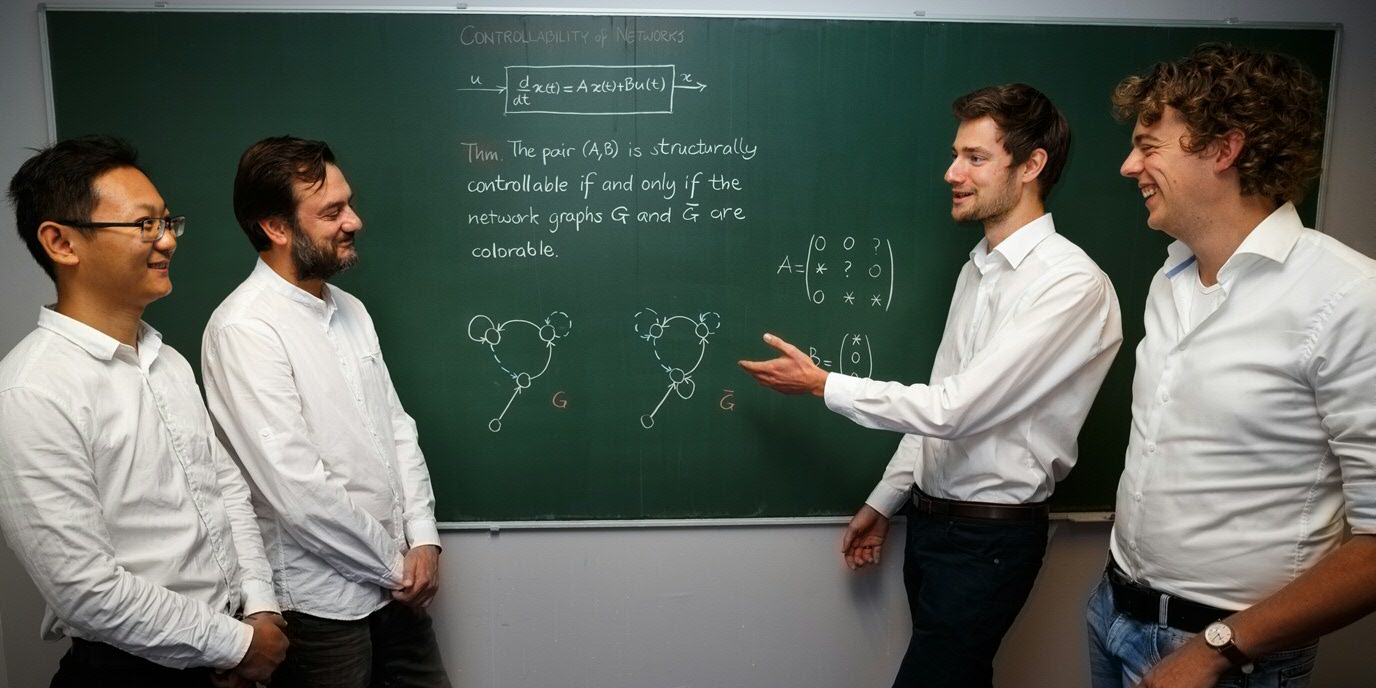
Systems, Control and Optimization | SCO
Our research group, within the Department of Mathematics, is part of the Bernoulli Institute for Mathematics, Computer Science and Artificial Intelligence (BI) of the University of Groningen, the Netherlands. The group is a member of Dutch Institute of Systems and Control as well as Jan C. Willems Center for Systems and Control.
Do you know about the Bernoulli Institutes Intranet pages as they contain very useful information benefitial for all Bernoulli staff and PhD's, please have a look.